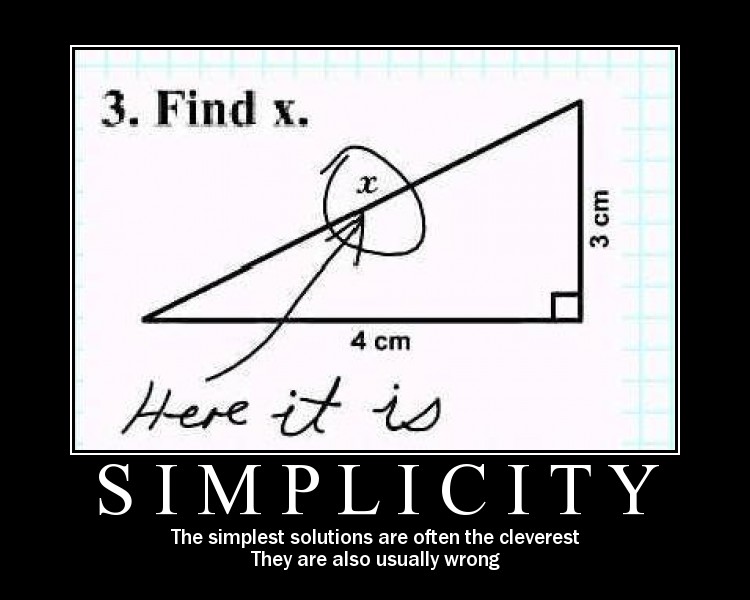
Motivational Posters Thread
- Thread starter Vagabond
- Start date
- Status
- Not open for further replies.
More options
Export threadSome that I had saved from the old boards.














figmentPez
Staff member
New ones!












Win. Epic, epic win.figmentPez said:
R
Rubicon
someones been reading 4chan todayDenbrought said:
Ross
Staff member
Only, the "Given a=b" part is a false, since it's impossible for a to equal b. Hence, while the algebra is correct, the initial assumption is incorrect.Allen said: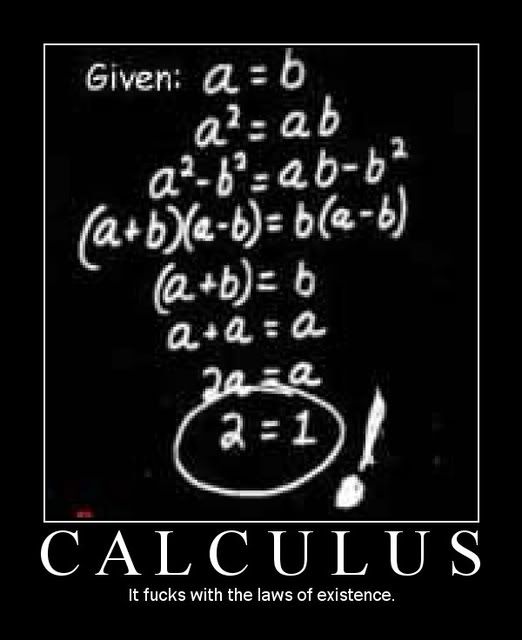
It's a pity the math is terrible.
2a=a is perfectly valid if a=0.Rasputin said:Only, the "Given a=b" part is a false, since it's impossible for a to equal b. Hence, while the algebra is correct, the initial assumption is incorrect.Allen said:
It's a pity the math is terrible.
therefore, it's obvious that dividing both sides by a (0) would produce a nonsensical result.
GasBandit
Staff member
You just divided by WHAT?!!Tinwhistler said:2a=a is perfectly valid if a=0.
therefore, it's obvious that dividing both sides by a (0) would produce a nonsensical result.

They also divide by zero when they divide to remove (a-b) since a-b=0Tinwhistler said:2a=a is perfectly valid if a=0.Rasputin said:Only, the "Given a=b" part is a false, since it's impossible for a to equal b. Hence, while the algebra is correct, the initial assumption is incorrect.Allen said:[bad math]
It's a pity the math is terrible.
therefore, it's obvious that dividing both sides by a (0) would produce a nonsensical result.
They also divide by zero when they divide to remove (a-b) since a-b=0[/quote:bxsoxely]Allen said:2a=a is perfectly valid if a=0.Tinwhistler said:Only, the "Given a=b" part is a false, since it's impossible for a to equal b. Hence, while the algebra is correct, the initial assumption is incorrect.Rasputin said:[quote="Allen, who is Quiet":bxsoxely][bad math]
It's a pity the math is terrible.
therefore, it's obvious that dividing both sides by a (0) would produce a nonsensical result.
Which is the main flaw in the deduction.
Also, of course, it isn't calculus, it's algebra.Allen said:
It's a pity the math is terrible.
I don't make them, I just post them.Dieb said:Also, of course, it isn't calculus, it's algebra.Allen said:It's a pity the math is terrible.
Ross
Staff member
I still stand by my previous post... That the assumption that a=b is invalid. As previously said, although it is possible at the end, a has to not be 0 in order to get to that point, therefore a=0 is not a viable answer.
The mathematicians on this forum SHOULD agree with me on this one... :|
The mathematicians on this forum SHOULD agree with me on this one... :|
a=b is a perfectly valid assumption. Variables can be equal to each other.Rasputin said:I still stand by my previous post... That the assumption that a=b is invalid. As previously said, although it is possible at the end, a has to not be 0 in order to get to that point, therefore a=0 is not a viable answer.
The mathematicians on this forum SHOULD agree with me on this one... :|
R
Rubicon
less math more posters?
a^2+b^2=c^2 in a right triangleMav said:less math more posters?
cos(-x)=cos(x)
sin(2x)=2sinxcosx
c^2=a^2+b^2-2abcosC
Prove: cos2y x secy=2cosy-secy
Given: cos2y x secy
=(2cos^2y-1) x secy
=(2cos^2y x secy)-secy
=(2cos^2y x 1/cosy)-secy
=2cosy-secy

W
wana10
as allen who is quiet said the problem isn't that a=b, which is perfectly valid. the problem is that for any value of a and b you will have to divide by zero and then...Rasputin said:I still stand by my previous post... That the assumption that a=b is invalid. As previously said, although it is possible at the end, a has to not be 0 in order to get to that point, therefore a=0 is not a viable answer.
The mathematicians on this forum SHOULD agree with me on this one... :|


figmentPez
Staff member
No, I'd rather not get too cozy or sexy with math. I want more posters!Allen said:=2cosy-secy

They also divide by zero when they divide to remove (a-b) since a-b=0[/quote:1mkfgykj]Allen said:2a=a is perfectly valid if a=0.Tinwhistler said:Only, the "Given a=b" part is a false, since it's impossible for a to equal b. Hence, while the algebra is correct, the initial assumption is incorrect.Rasputin said:[quote="Allen, who is Quiet":1mkfgykj][bad math]
It's a pity the math is terrible.
therefore, it's obvious that dividing both sides by a (0) would produce a nonsensical result.
This should have been posted first.
A
Anubinomicon
is this guy shirtless on a plane or bus? where is he leaving? Arkansas? Hey guy just make yourself comfortable, there's probably room to put your trans am up on cinderblocks in the backfigmentPez said:New ones!

Ross
Staff member
The problem isn't that they can't be equal. I'm saying that they ASSUME IN THE BEGINNING BEFORE THEY EVEN START that a=b AND IS TRUE. Through the rest of the problem, we know that a=b IS NOT true, and the assumption is therefore incorrect from the very start.Allen said:a=b is a perfectly valid assumption. Variables can be equal to each other.Rasputin said:I still stand by my previous post... That the assumption that a=b is invalid. As previously said, although it is possible at the end, a has to not be 0 in order to get to that point, therefore a=0 is not a viable answer.
The mathematicians on this forum SHOULD agree with me on this one... :|
It is an invalid assumption, since the assumption is that it is TRUE, when it is, in fact, FALSE.
EDIT:
While a can equal b in OTHER examples, it cannot in THIS example, which is what the whole argument is about.
GasBandit
Staff member
Math is all kinds of wack.Rasputin said:The problem isn't that they can't be equal. I'm saying that they ASSUME IN THE BEGINNING BEFORE THEY EVEN START that a=b AND IS TRUE. Through the rest of the problem, we know that a=b IS NOT true, and the assumption is therefore incorrect from the very start.Allen said:a=b is a perfectly valid assumption. Variables can be equal to each other.Rasputin said:I still stand by my previous post... That the assumption that a=b is invalid. As previously said, although it is possible at the end, a has to not be 0 in order to get to that point, therefore a=0 is not a viable answer.
The mathematicians on this forum SHOULD agree with me on this one... :|
It is an invalid assumption, since the assumption is that it is TRUE, when it is, in fact, FALSE.
x = 0.99999...
10x = 9.99999....
=> 9x = 9 (subtracting 1x from both sides of the equation)
=> x = 1
Oh, I'm sure you'll have nits to pick there, so let's do it this way...
1/3=0.333...
(multiply both sides by 3)
3/3=0.999...
3/3=1
1=0.999...
MAAAAJIIIIC!
GasBandit said:Math is all kinds of wack.Rasputin said:The problem isn't that they can't be equal. I'm saying that they ASSUME IN THE BEGINNING BEFORE THEY EVEN START that a=b AND IS TRUE. Through the rest of the problem, we know that a=b IS NOT true, and the assumption is therefore incorrect from the very start.Allen said:a=b is a perfectly valid assumption. Variables can be equal to each other.Rasputin said:I still stand by my previous post... That the assumption that a=b is invalid. As previously said, although it is possible at the end, a has to not be 0 in order to get to that point, therefore a=0 is not a viable answer.
The mathematicians on this forum SHOULD agree with me on this one... :|
It is an invalid assumption, since the assumption is that it is TRUE, when it is, in fact, FALSE.
x = 0.99999...
10x = 9.99999....
=> 9x = 9 (subtracting 1x from both sides of the equation)
=> x = 1
Oh, I'm sure you'll have nits to pick there, so let's do it this way...
1/3=0.333...
(multiply both sides by 3)
3/3=0.999...
3/3=1
1=0.999...
MAAAAJIIIIC!
These only work because the decimal is repeating. The more repeats you use, the more true it is that .99999999=1

We assume in your example, that the repeats go out infinitely, which means that we really do approach 1 if you have .9 with an infinite number of nines repeating..
If you don't use repeating decimals, we end up with something like this:
X = 0.99999
10x = 9.9999
10X - X = 9.9999 - 0.99999 (note that 10X without repeats is 9.9999 not 9.99999)
9X = 8.99991
X = 0.99999
Z
Zarvox
a can still be equal to b throughout the problem. The only thing wrong is they divide by 0 and get 1=2. a=b is still valid. If there's a problem in one step (in this case, from 3 to 4), that doesn't mean earlier steps are incorrect.Rasputin said:The problem isn't that they can't be equal. I'm saying that they ASSUME IN THE BEGINNING BEFORE THEY EVEN START that a=b AND IS TRUE. Through the rest of the problem, we know that a=b IS NOT true, and the assumption is therefore incorrect from the very start.
It is an invalid assumption, since the assumption is that it is TRUE, when it is, in fact, FALSE.
EDIT:
While a can equal b in OTHER examples, it cannot in THIS example, which is what the whole argument is about.
Example:
1+1=2
1=3
That's wrong. 1 doesn't equal 3. That means the math is wrong, not the assumption that 1+1=2 is wrong.
GasBandit
Staff member
They all are just antsy ever since 7 8 9.ElJuski said:Okay, I'm banning anyone who makes posts another math poster that gets all the number dorks in a tizzy -_-. Where's the FUNNY, DAMNIT
- Status
- Not open for further replies.